% Section 7.1.1
% Boyd & Vandenberghe, "Convex Optimization"
% Original by Lieven Vandenberghe
% Adapted for CVX by Argyris Zymnis - 01/31/06
%
% We consider a binary random variable y with prob(y=1) = p and
% prob(y=0) = 1-p. We assume that that y depends on a vector of
% explanatory variables u in R^n. The logistic model has the form
% p = exp(a'*u+b)/(1+exp(a'*u+b)), where a and b are the model parameters.
% We have m data points (u_1,y_1),...,(u_m,y_m).
% We can reorder the data so that for u_1,..,u_q the outcome is y = 1
% and for u_(q+1),...,u_m the outcome is y = 0. Then it can be shown
% that the ML estimate of a and b can be found by solving
%
% minimize sum_{i=1,..,q}(a'*u_i+b) - sum_i(log(1+exp(a'*u_i+b)))
%
% In this case we have m = 100 and the u_i are just scalars.
% The figure shows the data as well as the function
% f(x) = exp(aml*x+bml)/(1+exp(aml*x+bml)) where aml and bml are the
% ML estimates of a and b.

randn('state',0);
rand('state',0);

% Generate data
a =  1;
b = -5 ;
m= 100;

u = 10*rand(m,1);
y = (rand(m,1) < exp(a*u+b)./(1+exp(a*u+b)));
plot(u,y,'o')
axis([-1,11,-0.1, 1.1]);

% Solve problem
%
% minimize  -(sum_(y_i=1) ui)*a - b + sum log (1+exp(a*ui+b)

U = [ones(m,1) u];
cvx_expert true
cvx_begin
    variables x(2)
    maximize(y'*U*x-sum(log_sum_exp([zeros(1,m); x'*U'])))
cvx_end

% Plot results and logistic function

ind1 = find(y==1);
ind2 = find(y==0);

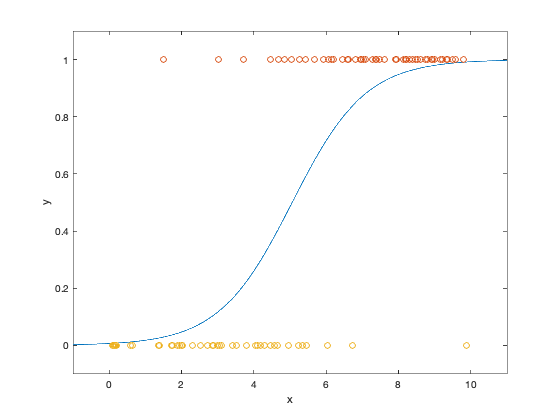
aml = x(2);  bml = x(1);
us = linspace(-1,11,1000)';
ps = exp(aml*us + bml)./(1+exp(aml*us+bml));

dots = plot(us,ps,'-', u(ind1),y(ind1),'o',...
     u(ind2),y(ind2),'o');

axis([-1, 11,-0.1,1.1]);
xlabel('x');
ylabel('y');

 
Calling Mosek 9.1.9: 600 variables, 202 equality constraints
   For improved efficiency, Mosek is solving the dual problem.
------------------------------------------------------------

MOSEK Version 9.1.9 (Build date: 2019-11-21 11:32:15)
Copyright (c) MOSEK ApS, Denmark. WWW: mosek.com
Platform: MACOSX/64-X86

Problem
  Name                   :                 
  Objective sense        : min             
  Type                   : CONIC (conic optimization problem)
  Constraints            : 202             
  Cones                  : 200             
  Scalar variables       : 600             
  Matrix variables       : 0               
  Integer variables      : 0               

Optimizer started.
Presolve started.
Linear dependency checker started.
Linear dependency checker terminated.
Eliminator started.
Freed constraints in eliminator : 0
Eliminator terminated.
Eliminator started.
Freed constraints in eliminator : 0
Eliminator terminated.
Eliminator - tries                  : 2                 time                   : 0.00            
Lin. dep.  - tries                  : 1                 time                   : 0.00            
Lin. dep.  - number                 : 0               
Presolve terminated. Time: 0.00    
Problem
  Name                   :                 
  Objective sense        : min             
  Type                   : CONIC (conic optimization problem)
  Constraints            : 202             
  Cones                  : 200             
  Scalar variables       : 600             
  Matrix variables       : 0               
  Integer variables      : 0               

Optimizer  - threads                : 8               
Optimizer  - solved problem         : the primal      
Optimizer  - Constraints            : 202
Optimizer  - Cones                  : 200
Optimizer  - Scalar variables       : 600               conic                  : 600             
Optimizer  - Semi-definite variables: 0                 scalarized             : 0               
Factor     - setup time             : 0.00              dense det. time        : 0.00            
Factor     - ML order time          : 0.00              GP order time          : 0.00            
Factor     - nonzeros before factor : 703               after factor           : 703             
Factor     - dense dim.             : 0                 flops                  : 1.03e+04        
ITE PFEAS    DFEAS    GFEAS    PRSTATUS   POBJ              DOBJ              MU       TIME  
0   3.4e+01  1.3e+00  2.1e+02  0.00e+00   2.130582563e+02   0.000000000e+00   1.0e+00  0.00  
1   6.1e+00  2.4e-01  2.5e+01  3.61e-01   2.485318230e+01   -3.026617046e+01  1.8e-01  0.01  
2   6.9e-01  2.7e-02  1.3e+00  6.57e-01   -2.492063045e+01  -3.259121601e+01  2.1e-02  0.01  
3   3.5e-02  1.4e-03  1.6e-02  9.13e-01   -3.239002363e+01  -3.279631727e+01  1.0e-03  0.01  
4   3.1e-03  1.2e-04  4.3e-04  9.93e-01   -3.292581875e+01  -3.296187533e+01  9.3e-05  0.01  
5   2.3e-04  8.7e-06  8.4e-06  1.00e+00   -3.297634919e+01  -3.297897289e+01  6.7e-06  0.01  
6   2.1e-05  7.9e-07  2.3e-07  1.00e+00   -3.297943954e+01  -3.297967826e+01  6.1e-07  0.02  
7   2.3e-06  9.0e-08  8.8e-09  1.00e+00   -3.297967636e+01  -3.297970359e+01  7.0e-08  0.02  
8   3.4e-07  1.3e-08  4.8e-10  1.00e+00   -3.297969912e+01  -3.297970306e+01  1.0e-08  0.02  
9   4.5e-08  1.7e-09  2.3e-11  1.00e+00   -3.297970220e+01  -3.297970272e+01  1.3e-09  0.02  
10  1.9e-08  7.2e-10  6.1e-12  1.00e+00   -3.297970246e+01  -3.297970268e+01  5.6e-10  0.02  
Optimizer terminated. Time: 0.02    


Interior-point solution summary
  Problem status  : PRIMAL_AND_DUAL_FEASIBLE
  Solution status : OPTIMAL
  Primal.  obj: -3.2979702463e+01   nrm: 1e+02    Viol.  con: 3e-07    var: 0e+00    cones: 0e+00  
  Dual.    obj: -3.2979702680e+01   nrm: 5e+00    Viol.  con: 0e+00    var: 0e+00    cones: 0e+00  
Optimizer summary
  Optimizer                 -                        time: 0.02    
    Interior-point          - iterations : 10        time: 0.02    
      Basis identification  -                        time: 0.00    
        Primal              - iterations : 0         time: 0.00    
        Dual                - iterations : 0         time: 0.00    
        Clean primal        - iterations : 0         time: 0.00    
        Clean dual          - iterations : 0         time: 0.00    
    Simplex                 -                        time: 0.00    
      Primal simplex        - iterations : 0         time: 0.00    
      Dual simplex          - iterations : 0         time: 0.00    
    Mixed integer           - relaxations: 0         time: 0.00    

------------------------------------------------------------
Status: Solved
Optimal value (cvx_optval): -32.9797